Unbiased test

A statistical test of size (level) 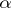, , 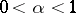for testing a compound hypothesis 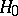: 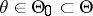against a compound alternative 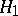: 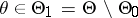, whose power function (cf. Power function of a test) satisfies

In many problems in statistical hypotheses testing there are no uniformly most-powerful tests (cf. Uniformly most-powerful test). But if one restricts the class of tests, then there may be uniformly most-powerful tests in that class. If in the problem of testing the hypothesis 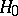against the alternative there is a uniformly most-powerful test, then it is unbiased (cf. Unbiased test), since the power of such a test cannot be less than that of the so-called trivial test whose critical function 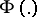is constant and equal to the size 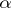, that is, , where 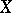is the random variable whose realization is used to test the hypothesis 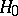against the alternative .

Example. The sign test is uniformly most-powerful unbiased in the problem of testing the hypothesis according to which the unknown true value of the parameter 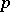of the binomial distribution is equal to 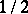against the alternative : .

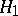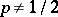References

[1]	E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1959)