Potential
potential function
A characteristic of a vector field.
A scalar potential is a scalar function 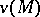 such that
such that 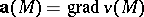 at every point of the domain of definition of the field
at every point of the domain of definition of the field  (sometimes, for example in physics, its negative is called a potential). If such a function exists, the vector field is called a potential field.
(sometimes, for example in physics, its negative is called a potential). If such a function exists, the vector field is called a potential field.
A vector potential is a vector function 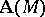 such that
such that  (cf. Curl) at every point of the domain of definition of the field
(cf. Curl) at every point of the domain of definition of the field  . If such a function exists, the vector field
. If such a function exists, the vector field  is called a solenoidal field.
is called a solenoidal field.
Depending on the distribution of the mass or the charge by which the potential is generated one speaks about a potential of a point-charge, a surface potential (single-layer or double-layer), a volume potential, etc. (see Potential theory).
Comments
See also Double-layer potential; Logarithmic potential; Multi-field potential; Newton potential; Non-linear potential; Riesz potential.
The use of a vector potential is restricted to three-dimensional vector fields. In this case one can prove the so-called Clebsch lemma, according to which any vector field can be represented as a sum of a potential field and a solenoidal field, 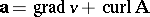 .
.
Potential. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Potential&oldid=42618