Arf-invariant

invariant of Arf

An invariant of a quadratic form modulo 2, given on an integral lattice endowed with a bilinear skew-symmetric form. Let 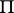be an integral lattice of dimension 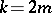and let 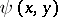be a form for which 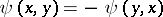. There exists bases 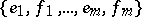, called symplectic bases, in which the matrix of reduces to block-diagonal form: The diagonal contains the blocks

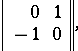i.e.

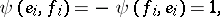while the other entries are zero.

Suppose that a mapping

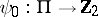is given on 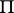such that

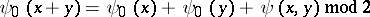(a "quadratic form modulo 2" ). The expression

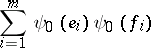is then called an Arf-invariant [1]. If this expression equals zero, then there is a symplectic basis on all elements of which 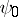vanishes; if this expression equals one, then there is a symplectic basis on all elements of which, except 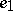and 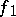, the form vanishes, while

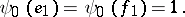References

[1]	C. Arf, "Untersuchungen über quadratischen Formen in Körpern der Charakteristik 2, I" J. Reine Angew. Math. , 183 (1941) pp. 148–167

Comments

See [a1], Appendix 1, for material concerning the Arf-invariant for inner product spaces over a field 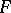of characteristic 2 in relation to the Witt algebra of quadratic inner product spaces over .

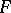References

[a1]	J. Milnor, D. Husemoller, "Symmetric bilinear forms" , Springer (1973)