Inversion

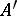A transformation taking each point of the plane to the point on the ray 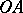for which 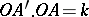, where 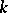is a constant real number. The point 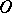is called the centre, or pole, of the inversion and 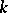the power, or coefficient, of the inversion. If , then points on the circle 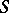with centre 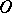and radius are taken to themselves under the inversion; interior points of are taken to exterior points and vice versa (an inversion is sometimes called a symmetry with respect to a circle). The centre of an inversion does not have an image. An inversion with negative power 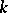is equivalent to the inversion with the same centre 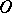and positive power followed by symmetry in . An inversion with positive power is sometimes called a hyperbolic inversion, while one with negative power is called an elliptic inversion or anti-inversion. A straight line passing through the centre of an inversion is taken into itself under the inversion. A straight line not passing through the centre of an inversion is taken into a circle passing through the centre of the inversion. A circle passing through the centre of an inversion is taken into a straight line not passing through the centre of the inversion. A circle not passing through the centre of an inversion is taken into a circle not passing through the centre of the inversion. In rectangular Cartesian coordinates an inversion can be given by:

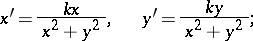and in the complex plane by the formula 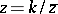. An inversion is an anti-conformal mapping, that is, it preserves angles between lines and changes their orientation. An inversion in space is defined in a similar way.

An inversion is sometimes defined as a mapping of the plane that associates with each point 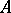distinct from the centre of a given pencil of circles the point of intersection 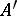of the circles of the pencil passing through .

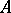References

[1]	P.S. Alexandroff [P.S. Aleksandrov] (ed.) et al. (ed.) , Enzyklopaedie der Elementarmathematik , 4. Geometrie , Deutsch. Verlag Wissenschaft. (1969) (Translated from Russian)
[2]	B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1976) (In Russian)

Comments

Sometimes an ideal point 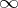is regarded as the image of the centre of an inversion under this inversion, especially when one considers the inversion on the extended complex plane .

References

[a1]	H. Schwerdtfeger, "Geometry of complex numbers" , Dover, reprint (1979)
[a2]	D. Pedoe, "Circles" , Pergamon (1957)