Pro-p group
A profinite group that is a projective limit of finite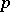 
-groups (cf. 
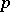-group). E.g. the additive group of the ring 
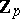of 
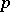-adic integers is a pro-
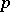-group. In Galois theory pro-
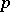-groups appear as Galois groups of 
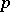-extensions of fields.
Let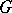 
be a pro-
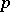-group. A system of generators of 
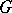is a subset 
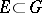with the properties: 1) 
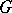is the smallest closed subgroup of 
generated by 
; and 2) any neighbourhood of the identity of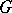 
contains almost-all (i.e. all except a finite number of) elements of 
.
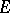Let 
be an index set and let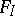 
be the abstract free group with system of generators 
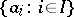. The projective limit 
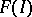of the system of groups 
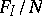, where 
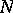is a normal subgroup of 
such that the index of 
in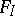 
is a power of a number 
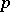, while almost-all elements 
, 
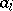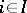, lie in 
, is a pro-
-group, called the free pro-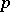
-group with system of generators 
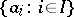. Every closed subgroup of a free pro-
-group is itself a free pro-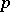
-group. Any pro-
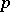-group 
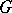is a quotient group of a free pro-
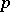-group, i.e. there is an exact sequence of homomorphisms of pro-
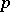-groups,
 |
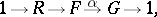where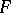 
is a suitable free pro-
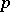-group. (This sequence is called a presentation of 
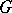by means of 
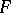.) A subset 
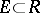is called a system of relations of 
if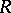 
is the smallest closed normal subgroup in 
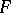containing 
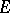and if every open normal subgroup in 
contains almost-all elements of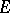 
. The cardinalities of a minimal (with respect to inclusion) set of generators and a minimal system of relations of a corresponding presentation of a pro-
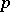-group 
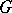have a cohomological interpretation: The first cardinality is the dimension over 
of the space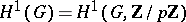 
, while the second is the dimension over 
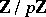of the space 
. Here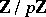 
is regarded as a discrete 
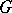-module with a trivial 
-action. If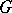 
is a finite 
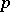-group, then
 |
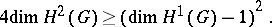This result implies a negative solution to the classical class field tower problem (cf. Tower of fields) [4].
References
| [1] | J.-P. Serre, "Cohomologie Galoisienne" , Springer (1964) |
| [2] | H. Koch, "Galoissche Theorie der  -Erweiterungen" , Deutsch. Verlag Wissenschaft. (1970) |
| [3] | J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1967) |
| [4] | E.S. Golod, I.R. Shafarevich, "On the class field tower" Izv. Akad. Nauk SSSR Ser. Mat. , 28 : 2 (1964) pp. 261–272 (In Russian) |
Pro-p group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pro-p_group&oldid=42149