Period of a function
A number 
such that for any 
(or 
) the numbers 
and 
also belong to 
and such that the following equality holds:
 |
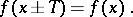The numbers 
, where 
is a natural number, are also periods of 
. For a function 
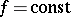on an axis or on a plane, any number 
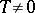is a period; for the Dirichlet function
 |
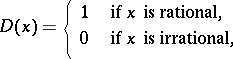any rational number 
is a period. If a function 
has period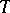 
, then the function 
, where 
and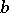 
are constants and 
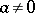, has period 
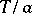. If a real-valued function 
of a real argument is periodic on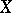 
(and is not identically equal to a constant), then it has a least period 
and any other real period is a multiple of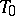 
. There exist non-constant functions of a complex argument having two non-multiple periods with imaginary quotient, such as for example an elliptic function.
Similarly one defines the period of a function defined on an Abelian group.
Comments
Cf. also Periodic function.
Period of a function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Period_of_a_function&oldid=42145