Algebraic space

A generalization of the concepts of a scheme and an algebraic variety. This generalization is the result of certain constructions in algebraic geometry: Hilbert schemes, Picard schemes, moduli varieties, contractions, which are often not realizable in the category of schemes and require its extensions. However, the category of algebraic spaces is closed under these constructions, which renders the algebraic space a natural object of algebraic geometry.

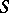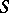Any scheme defines some sheaf in the étale topology of the category of schemes, which in turn uniquely defines the scheme . An algebraic space is a sheaf of sets 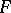in the étale topology of schemes satisfying the condition of local representability (in the étale topology): There exists a scheme 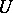and a sheaf morphism 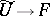such that for any scheme 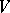and morphism 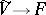the fibred product 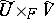is represented by a scheme 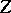, and the induced morphism of schemes 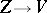is a surjective étale morphism. The scheme is known as the étale covering of the sheaf 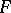, which is a quotient sheaf of the sheaf 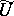according to the étale equivalence relation 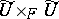. This last proposition reveals the geometric sense of an algebraic space as a quotient scheme according to the étale equivalence relation. Morphisms of an algebraic space are defined as morphisms of sheaves; the category of schemes becomes identical with a complete subcategory of the category of algebraic spaces.

Many concepts in the theory of schemes are applicable to algebraic spaces: point, local ring, étale topology, Zariski topology, field of functions, structure sheaf and coherent sheaves. Many results in the theory of schemes such as Serre's affinity criterion (cf. Affine scheme), and the theorem of finiteness and existence of a proper morphism, can be applied to algebraic spaces.

Finer results include the representability of Picard and Hilbert functors in the category of algebraic spaces. If a flat equivalence relation is given on an algebraic space, then the factorization by this relation yields an algebraic space (such a situation occurs, for example, when there is a free action of a finite group on the space). Finally, an algebraic space permits a contraction of a subspace with an ample conormal sheaf.

All algebraic spaces contain a subspace that is open and dense in the Zariski topology and that is a scheme. One-dimensional and non-singular two-dimensional algebraic spaces are schemes, but this is not true of three-dimensional or singular two-dimensional algebraic spaces; a group in the category of algebraic spaces over a field is a scheme. Complete algebraic spaces of dimension over the field of complex numbers have the natural structure of a compact analytic space with algebraically-independent meromorphic functions.

References

[1]	M. Artin, "Algebraic spaces" , Yale Univ. Press (1969) MR0427316 MR0407012 Zbl 0232.14003 Zbl 0226.14001 Zbl 0216.05501
[2]	D. Knutson, "Algebraic spaces" , Springer (1971) MR0302647 Zbl 0221.14001

Comments

The notion of an algebraic space was introduced by M. Artin and, in a slightly different but equivalent form, it was studied by B. Moishezon [a1]. The theorem cited at the end of the article (on subspaces) is due to him.

References

[a1]	B. Moishezon, "Algebraic varieties and compact complex spaces" , Proc. Internat. Congress Mathematicians (Nice, 1970) , 2 , Gauthier-Villars (1971) pp. 643–648 MR0425189 Zbl 0232.14004