Christoffel symbol
of a differential quadratic form
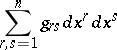 |
An abbreviated notation for the expression
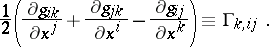 |
The symbols  are called the Christoffel symbols of the first kind, in contrast to the Christoffel symbols of the second kind,
are called the Christoffel symbols of the first kind, in contrast to the Christoffel symbols of the second kind,  , defined by
, defined by
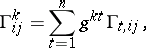 |
where  is defined as follows:
is defined as follows:
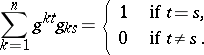 |
These symbols were introduced by E.B. Christoffel in 1869.
Comments
Let  ,
,  , be a linear connection on a manifold
, be a linear connection on a manifold  , where
, where  denotes the space of vector fields on
denotes the space of vector fields on  . Let
. Let  be a chart of
be a chart of  . Then on
. Then on  ,
,  is completely determined by
is completely determined by 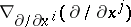 , where
, where  are coordinates on
are coordinates on  . The Christoffel symbols of the connection
. The Christoffel symbols of the connection  are now given by
are now given by
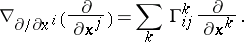 |
It is important to note that the 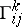 are not the components of a tensor field. In fact if the
are not the components of a tensor field. In fact if the  denote the Christoffel symbols of
denote the Christoffel symbols of  with respect to a second set of coordinates
with respect to a second set of coordinates  on
on  , then
, then
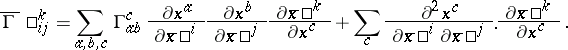 |
Let now  be the Riemannian connection (cf. Riemannian geometry) defined by a (local) Riemannian metric
be the Riemannian connection (cf. Riemannian geometry) defined by a (local) Riemannian metric  . Then the Christoffel symbols of this quadratic differential form are those of the connection
. Then the Christoffel symbols of this quadratic differential form are those of the connection  . I.e.,
. I.e.,
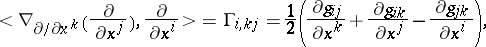 |
so that indeed
 |
where the 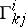 are the Christoffel symbols of the second kind of the quadratic differential form as defined above.
are the Christoffel symbols of the second kind of the quadratic differential form as defined above.
References
| [a1] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1 , Interscience (1963) pp. Chapt. 4 |
| [a2] | R.S. Millman, G.D. Parker, "Elements of differential geometry" , Prentice-Hall (1977) pp. Chapt. 7 |
Christoffel symbol. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Christoffel_symbol&oldid=41547