Wiener Tauberian theorem

If 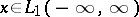has nowhere vanishing Fourier transform and 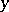is a function in 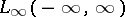such that the convolution 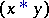tends to zero as , then the convolution , for any 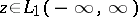tends to zero as . Established by N. Wiener [1]. This theorem was generalized to include any commutative locally compact non-compact group 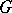: If is a function on , summable with respect to the Haar measure, whose Fourier transform does not vanish on the group of characters 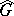of 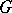and if is a function in 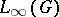such that the convolution 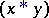tends to zero at infinity on 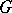, then the convolution tends to zero at infinity on 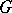for all summable functions on .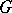

This theorem is based on the regularity of the group algebra of a commutative locally compact group, and on the possibility of spectral synthesis in group algebras for closed ideals belonging to only a finite number of regular maximal ideals [3].

References

[1]	N. Wiener, "Tauberian theorems" Ann. of Math. (2) , 33 : 1 (1932) pp. 1–100
[2]	M.A. Naimark, "Normed rings" , Reidel (1984) (Translated from Russian)
[3]	N. Bourbaki, "Théories spectrales" , Eléments de mathématiques , Hermann (1967)

References

[a1]	E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , 2 , Springer (1970)
[a2]	W. Rudin, "Fourier analysis on groups" , Interscience (1962)
[a3]	H. Reiter, "Classical harmonic analysis and locally compact groups" , Clarendon Press (1968)