Total set
From Encyclopedia of Mathematics
A set 
of linear functionals on a vector space 
separating the points of 
, that is, such that for any non-zero vector 
there is an 
with 
.
Comments
A total set in the sense above is also, and more precisely, called a total set of linear functions, [a1].
More generally, a set 
, where 
is a topological vector space, is called a total set or fundamental set if the linear span of 
is dense in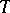 
. If 
, the algebraic dual of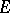 
, is given the weak topology (so that 
, if 
is the base field and 
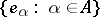is an (algebraic) basis for 
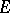), the two definitions for a set 
agree.
References
| [a1] | S. Rolewicz, "Metric linear spaces" , Reidel (1985) pp. 44 |
| [a2] | G. Köthe, "Topological vector spaces" , 1 , Springer (1969) pp. 132, 247ff |
How to Cite This Entry:
Total set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Total_set&oldid=39955
Total set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Total_set&oldid=39955
This article was adapted from an original article by V.I. Lomonosov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article