Wallman compactification

Wallman–Shanin compactification, 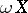, of a topological space 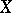satisfying the axiom 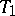(cf. Separation axiom)

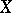The space whose points are maximal centred systems of closed sets in (cf. Centred family of sets). The topology in is given by the closed base 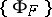, where ranges over all closed sets in 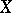and 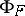consists of precisely those 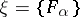for which for some .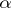

This compactification was described by H. Wallman [1].

The Wallman compactification is always a compact 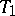-space; for a normal space it coincides with the Stone–Čech compactification.

If in defining the extension one chooses not all closed sets, but only those contained in a certain fixed closed base, one obtains a so-called compactification of Wallman type. Not every Hausdorff compactification of a Tikhonov space is a compactification of Wallman type.

References

[1]	H. Wallman, "Lattices and topological spaces" Ann of Math. , 39 (1938) pp. 112–126

Comments

Compactifications that are not Wallman compactifications were constructed by V.M. Ul'yanov [a1].

References

[a1]	V.M. Ul'yanov, "Solution of a basic problem on compactifications of Wallman type" Soviet Math. Dokl. , 18 (1977) pp. 567–571 Dokl. Akad. Nauk SSSR , 233 : 6 (1977) pp. 1056–1059
[a2]	R.A. Alo, H.L. Shapiro, "Normal bases and compactifications" Math. Ann. , 175 (1968) pp. 337–340
[a3]	O. Frink, "Compactifications and semi-normal spaces" Amer. J. Math. , 86 (1964) pp. 602–607
[a4]	R.C. Walker, "The Stone–Čech compactification" , Springer (1974)