Ramanujan hypothesis

Ramanujan conjecture

The conjecture, stated by S. Ramanujan [1], that the Fourier coefficients 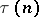of the function 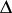(a cusp form of weight 12) satisfy the inequality

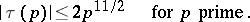is also called the Ramanujan function. The function 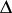is an eigen function of the Hecke operator, and the 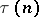are the corresponding eigen values. H. Petersson generalized Ramanujan's hypothesis to the case of eigen values of the Hecke operators on modular forms of weight 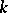, 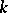an integer (the Petersson conjecture). P. Deligne (see [2]) reduced the Petersson conjecture to the Weil conjectures (cf. Zeta-function), then proved the latter (1974). This also proved Ramanujan's hypothesis.

References

[1]	S. Ramanujan, "On certain arithmetical functions" Trans. Cambridge Philos. Soc. , 22 (1916) pp. 159–184
[2]	P. Deligne, "La conjecture de Weil 1" Publ. Math. IHES , 43 (1974) pp. 273–307
[3]	O.M. Fomenko, "Applications of the theory of modular forms to number theory" J. Soviet Math. , 14 : 4 (1980) pp. 1307–1362 Itogi Nauk. i Tekhn. Algebra Topol. Geom. , 15 (1977) pp. 5–91

Comments

See also Congruence equation.

References

[a1]	N.M. Katz, "An overview of Deligne's proof of the Riemann hypothesis for varieties over finite fields" F.E. Browder (ed.) , Mathematical developments arising from Hilbert problems , Proc. Symp. Pure Math. , 28 , Amer. Math. Soc. (1976) pp. 275–305