Submodule

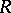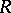A subset of a module which is a subgroup of its additive group and which is closed under multiplication by elements of the ground ring. In particular, a left (right) ideal of a ring is a submodule of the left (right) -module . A submodule distinct from the module itself and the zero submodule is called proper. The set of submodules of a given module, ordered by inclusion, is a complete Dedekind lattice (see Completely-reducible module). If 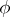is a homomorphism from a module 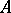into a module 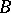, then the set

is a submodule of , called the kernel of the homomorphism 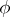. Each submodule is the kernel of some homomorphism. A submodule is called essential (large or principal) if its intersection with any other non-zero submodule is non-zero. For example, the integers form an essential submodule of the group of rational numbers. Each module is an essential submodule of its injective envelope (see Injective module). A submodule 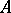of a module 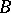is called inessential (small or co-principal) if for any submodule the equation 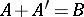implies . Any proper submodule of a chain module is inessential. For example, the non-invertible elements in a local ring form an inessential submodule. The sum of all inessential submodules coincides with the intersection of all maximal submodules. A left ideal 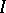belongs to the Jacobson radical if and only if 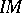is inessential in 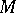for any finitely-generated left module . The elements of a small submodule are non-generating, i.e. any system of generators for a module remains generating after the removal of any of the elements (this of course does not mean that they can all be removed at once). The Jacobson radical of the endomorphism ring of a module coincides with the set of endomorphisms having an inessential image.

References

[1]	F. Kasch, "Modules and rings" , Acad. Press (1982) (Translated from German)
[2]	C. Faith, "Algebra" , 1–2 , Springer (1973–1976)