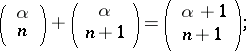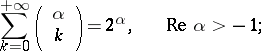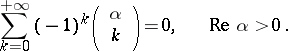The coefficients at the powers of  in the decomposition of the Newton binomial
in the decomposition of the Newton binomial 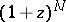 . The binomial coefficients are denoted by
. The binomial coefficients are denoted by
or by 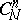 , and are given by
, and are given by
 | (1) |
The first-mentioned notation is due to L. Euler; the notation 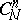 appeared in the 19th century, and is probably connected with the interpretation of the binomial coefficients
appeared in the 19th century, and is probably connected with the interpretation of the binomial coefficients 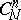 as the number of distinguishable non-ordered combinations (cf. Combination) from
as the number of distinguishable non-ordered combinations (cf. Combination) from  different objects with
different objects with  objects in each combination. Binomial coefficients are most conveniently written as numbers in the arithmetical triangle, or Pascal triangle, the construction of which is based on the following property of binomial coefficients:
objects in each combination. Binomial coefficients are most conveniently written as numbers in the arithmetical triangle, or Pascal triangle, the construction of which is based on the following property of binomial coefficients:
 | (2) |
Binomial coefficients, as well as the arithmetical triangle, were known concepts to the mathematicians of antiquity, in more or less developed forms. B. Pascal (l665) conducted a detailed study of binomial coefficients. The binomial coefficients are also connected by many useful relationships other than (2), for example:
 | (3a) |
 | (3b) |
 | (3c) |
 | (3d) |
 | (3e) |
 | (3f) |
In particular, (3a)–(3f) yields
 | (4) |
The use of the Stirling formula yields approximate expressions for binomial coefficients. Thus, if  is much larger than
is much larger than  :
:
In the case of a complex number 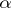 , binomial coefficients are generalized according to the formula
, binomial coefficients are generalized according to the formula
In this generalization, some of the relations (2)–(4) are preserved, but usually in a modified form. For instance,
For tables of binomial coefficients see [2], [3].
References
| [1] | G.A. Korn, T.M. Korn, "Mathematical handbook for scientists and engineers" , McGraw-Hill (1968) |
| [2] | L.N. Bol'shev, N.V. Smirnov, "Tables of mathematical statistics" , Libr. math. tables , 46 , Nauka (1983) (In Russian) (Processed by L.S. Bark and E.S. Kedrova) |
| [3] | , Table of binomial coefficients , Cambridge, Mass. (1954) |
How to Cite This Entry:
Binomial coefficients. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Binomial_coefficients&oldid=39155
This article was adapted from an original article by E.D. Solomentsev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article in the decomposition of the Newton binomial
in the decomposition of the Newton binomial 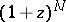 . The binomial coefficients are denoted by
. The binomial coefficients are denoted by
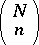
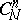 , and are given by
, and are given by

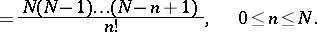
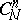 appeared in the 19th century, and is probably connected with the interpretation of the binomial coefficients
appeared in the 19th century, and is probably connected with the interpretation of the binomial coefficients 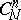 as the number of distinguishable non-ordered combinations (cf. Combination) from
as the number of distinguishable non-ordered combinations (cf. Combination) from  different objects with
different objects with  objects in each combination. Binomial coefficients are most conveniently written as numbers in the arithmetical triangle, or Pascal triangle, the construction of which is based on the following property of binomial coefficients:
objects in each combination. Binomial coefficients are most conveniently written as numbers in the arithmetical triangle, or Pascal triangle, the construction of which is based on the following property of binomial coefficients:









 is much larger than
is much larger than  :
:

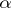 , binomial coefficients are generalized according to the formula
, binomial coefficients are generalized according to the formula