Lebesgue dimension
A dimension defined by means of coverings (cf. Covering (of a set)). It is the most important dimension invariant  of a topological space
of a topological space  and was discovered by H. Lebesgue [1]. He stated the conjecture that
and was discovered by H. Lebesgue [1]. He stated the conjecture that  for the
for the  -dimensional cube
-dimensional cube  . L.E.J. Brouwer [2] was the first to prove this, as well as the stronger identity:
. L.E.J. Brouwer [2] was the first to prove this, as well as the stronger identity:  . A precise definition of the invariant
. A precise definition of the invariant  (for the class of metric compacta) was given by P.S. Urysohn, who proved that for a space
(for the class of metric compacta) was given by P.S. Urysohn, who proved that for a space  of this class
of this class
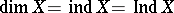 |
(the Urysohn identity, see Dimension theory). This identity was extended to the class of all separable metric spaces by W. Hurewicz and L.A. Tumarkin in 1925.
For compacta  the Lebesgue dimension is defined as the smallest integer
the Lebesgue dimension is defined as the smallest integer  having the property that for any
having the property that for any 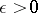 there is a finite open
there is a finite open  -covering of
-covering of  that has multiplicity
that has multiplicity 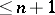 ; an
; an  -covering of a metric space is a covering all elements of which have diameter
-covering of a metric space is a covering all elements of which have diameter  , and the multiplicity of a finite covering of
, and the multiplicity of a finite covering of 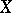 is the largest integer
is the largest integer  such that there is a point of
such that there is a point of  contained in
contained in 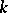 elements of the given covering. For an arbitrary normal (in particular, metrizable) space
elements of the given covering. For an arbitrary normal (in particular, metrizable) space 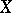 the Lebesgue dimension is the smallest integer
the Lebesgue dimension is the smallest integer  such that for any finite open covering
such that for any finite open covering 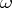 of
of  there is a (finite open) covering
there is a (finite open) covering 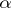 of multiplicity
of multiplicity 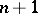 that refines it. A covering
that refines it. A covering  is said to be a refinement of a covering
is said to be a refinement of a covering  if every element of
if every element of 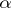 is a subset of at least one element of
is a subset of at least one element of 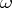 .
.
References
| [1] | H. Lebesgue, "Sur la non-applicabilité de deux domaines appartenant à des espaces à  et et 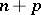 dimensions" Math. Ann. , 70 (1911) pp. 166–168 dimensions" Math. Ann. , 70 (1911) pp. 166–168 |
| [2] | L.E.J. Brouwer, "Ueber den natürlichen Dimensionsbegriff" J. Reine Angew. Math. , 142 (1913) pp. 146–152 |
| [3] | P.S. Aleksandrov, B.A. Pasynkov, "Introduction to dimension theory" , Moscow (1973) (In Russian) |
Comments
The Lebesgue dimension is also called the covering dimension or Čech–Lebesgue dimension. The multiplicity of a covering is also called the order of the covering.
References
| [a1] | R. Engelking, "Dimension theory" , North-Holland & PWN (1978) pp. 19; 50 |
| [a2] | W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) ((Appendix by L.S. Pontryagin and L.G. Shnirel'man in Russian edition.)) |
Lebesgue dimension. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lebesgue_dimension&oldid=38632