Keldysh-Lavrent'ev theorem
on uniform approximation by entire functions
In order that there exist for any continuous complex-valued function  on a continuum
on a continuum 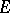 and any rapidly-decreasing positive function
and any rapidly-decreasing positive function  ,
,  (as
(as  ), having a positive lower bound on any finite interval, an entire function
), having a positive lower bound on any finite interval, an entire function  such that
such that
 |
it is necessary and sufficient that 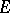 has no interior points and that there exists a function
has no interior points and that there exists a function  ,
,  , that increases to
, that increases to  and is such that any point
and is such that any point  of the complement
of the complement  can be joined to
can be joined to 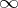 by a Jordan curve situated outside
by a Jordan curve situated outside  and outside the disc
and outside the disc 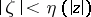 .
.
This result of M.V. Keldysh and M.A. Lavrent'ev [1] summarizes numerous investigations on approximation by entire functions initiated by the Carleman theorem (Section 3; see also [2]).
References
| [1] | M.V. Keldysh, M.A. Lavrent'ev, "Sur un problème de M. Carleman" Dokl. Akad. Nauk SSSR , 23 : 8 (1939) pp. 746–748 |
| [2] | S.N. Mergelyan, "Uniform approximation to functions of a complex variable" Transl. Amer. Math. Soc. , 3 (1962) pp. 294–391 Uspekhi Mat. Nauk , 7 : 2 (1952) pp. 31–1A2 |
Comments
References
| [a1] | D. Gaier, "Lectures on complex approximation" , Birkhäuser (1987) (Translated from German) |
Keldysh-Lavrent'ev theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Keldysh-Lavrent%27ev_theorem&oldid=36636