Hilbert geometry

The geometry of a complete metric space 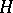with a metric 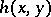which contains, together with two arbitrary, distinct points and , also the points and 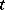for which , , 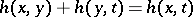and which is homeomorphic to a convex set in an -dimensional affine space , the geodesics being mapped to straight lines of 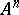. 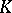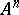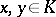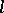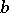Thus, let be a convex body in with boundary not containing two non-collinear segments, and let be located on a straight line which intersects at and ; let 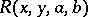be the cross ratio of , , 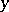, (so that if 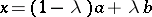, , 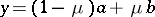then 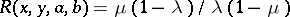). Then

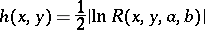is the metric of a Hilbert geometry (a Hilbert metric). If 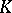is centrally symmetric, then 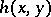is a Minkowski metric (cf. Minkowski geometry); if 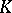is an ellipsoid, then 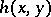defines the Lobachevskii geometry.

The problem of determining all metrizations of for which the geodesics are straight lines is Hilbert's fourth problem; it has been completely solved [4].

Geodesic geometry is a generalization of Hilbert geometry.

Hilbert geometry was first mentioned in 1894 by D. Hilbert in a letter to F. Klein.

References

[1]	D. Hilbert, "Grundlagen der Geometrie" , Springer (1913)
[2]	"Hilbert problems" Bull. Amer. Math. Soc. , 8 (1902) pp. 437–479 (Translated from German)
[3]	H. Busemann, "The geometry of geodesics" , Acad. Press (1955)
[4]	A.V. Pogorelov, "Hilbert's fourth problem" , Winston & Wiley (1974) (In Russian)

References

[a1]	H. Busemann, P.J. Kelly, "Projective geometry and projective metrics" , Acad. Press (1953)
[a2]	M. Berger, "Geometry" , I , Springer (1987)