Symmetric polynomial

A polynomial 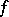, with coefficients in a field or a commutative associative ring 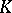with a unit, which is a symmetric function in its variables, that is, is invariant under all permutations of the variables:

(*)

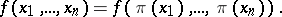The symmetric polynomials form the algebra over .

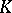The most important examples of symmetric polynomials are the elementary symmetric polynomials

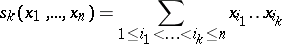and the power sums

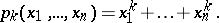The latter can be expressed in terms of elementary symmetric polynomials by recurrence formulas, called Newton's formulas:

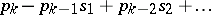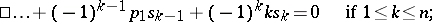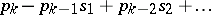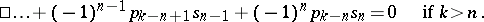For the elementary symmetric polynomials () of the roots of an arbitrary polynomial in one variable with leading coefficient 1, 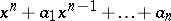, one has 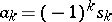(see Viète theorem).

The fundamental theorem on symmetric polynomials: Every symmetric polynomial is a polynomial in the elementary symmetric polynomials, and this representation is unique. In other words, the elementary symmetric polynomials are a set of free generators for the algebra 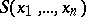. If the field has characteristic 0, then the polynomials also form a set of free generators of this algebra.

A skew-symmetric, or alternating, polynomial is a polynomial 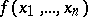satisfying the relation (*) if 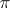is even and the relation

if 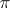is odd. Any skew-symmetric polynomial can be written in the form 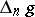, where 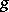is a symmetric polynomial and

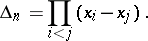This representation is not unique, in view of the relation .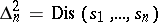

References

[1]	A.G. Kurosh, "Higher algebra" , MIR (1972) (Translated from Russian)
[2]	A.I. Kostrikin, "Introduction to algebra" , Springer (1982) (Translated from Russian)
[3]	A.P. Mishina, I.V. Proskuryakov, "Higher algebra. Linear algebra, polynomials, general algebra" , Pergamon (1965) (Translated from Russian)

Comments

Another important set of symmetric polynomials, which appear in the representations of the symmetric group, are the Schur polynomials (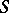-functions) 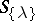. These are defined for any partition 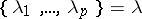, and include as special cases the above functions, e.g. 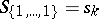, 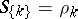(see, e.g., [a4], Chapt. VI).

In general, the discriminant of the polynomial 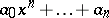with roots is defined as 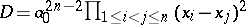, and satisfies

with .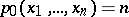

See Discriminant.

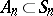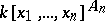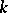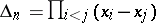Let be the alternating group, consisting of the even permutations. The ring of polynomials of polynomials over a field obviously contains the elementary symmetric functions and . If 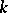is not of characteristic , the ring of polynomials is generated by and 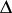, and the ideal of relations is generated by 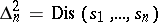. The condition is also necessary for the statement that every skew-symmetric polynomial is of the form 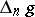with symmetric. More precisely, what is needed for this is that 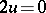implies 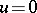for .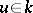

References

[a1]	N. Jacobson, "Basic algebra" , 1 , Freeman (1974)
[a2]	A.G. Kurosh, "An introduction to algebra" , MIR (1971) (Translated from Russian)
[a3]	B.L. van der Waerden, "Algebra" , 1 , Springer (1967) (Translated from German)
[a4]	D.E. Littlewood, "The theory of group characters and matrix representations of groups" , Clarendon Press (1950)
[a5]	V. Poénaru, "Singularités en présence de symmétrie" , Springer (1976) pp. 14ff
[a6]	P.M. Cohn, "Algebra" , 1 , Wiley (1982) pp. 181