Holomorphic envelope

envelope of holomorphy, of a (Riemann) domain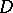

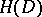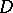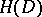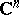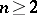The largest domain with the following property: Any holomorphic function in can be holomorphically continued to . The problem of constructing the envelope of holomorphy for a given domain arises in connection with the fact that in a complex space , , not all domains are domains of holomorphy (cf. Domain of holomorphy), i.e. there exist domains such that any function that is holomorphic in this domain has a holomorphic continuation to a larger (usually not single-layered) domain. The envelope of holomorphy 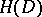is a domain of holomorphy; if 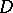is a domain of holomorphy, then .

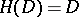In applications in axiomatic quantum field theory there arises the non-trivial problem of constructing envelopes of holomorphy of a special kind, which reflect the physical requirements of spectrality, local commutativity and Lorentz covariance. The Bogolyubov theorem on the edge-of-the-wedge and continuity theorems (cf. Continuity theorem) are especially useful in this connection.

References

[1]	V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian)
[2]	B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1976) (In Russian)

References

[a1]	R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Sect. G