Divisibility in rings

A generalization of the concept of divisibility of integers without remainder (cf. Division).

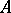An element of a ring is divisible by another element 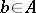if there exists a such that 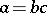. One also says that divides and is said to be a multiple of , while 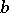is the divisor of . The divisibility of by 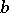is denoted by the symbol .

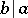Any associative-commutative ring displays the following divisibility properties:

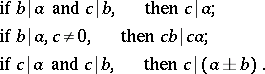The last two properties are equivalent to saying that the set of elements divisible by forms an ideal, , of the ring 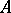(the principal ideal generated by the element 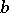), which contains 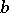if 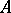is a ring with a unit element.

In an integral domain, elements and 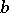are simultaneously divisible by each other ( 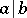and 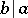) if and only if they are associated, i.e. 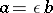, where is an invertible element. Two associated elements generate the same principal ideal. The unit divisors coincide, by definition, with invertible elements. A prime element in a ring is a non-zero element without proper divisors except unit divisors. In the ring of integers such elements are called primes (or prime numbers), and in a ring of polynomials they are known as irreducible polynomials. Rings in which — like in rings of integers or polynomials — there is unique decomposition into prime factors (up to unit divisors and the order of the sequence) are called factorial rings. For any finite set of elements in such a ring there exists a greatest common divisor and a lowest common multiple, both these quantities being uniquely determined up to unit divisors.

References

[1]	E. Kummer, "Zur Theorie der komplexen Zahlen" J. Reine Angew. Math. , 35 (1847) pp. 319–326
[2]	I.M. Vinogradov, "Elements of number theory" , Dover, reprint (1954) (Translated from Russian)
[3]	Z.I. Borevich, I.R. Shafarevich, "Number theory" , Acad. Press (1966) (Translated from Russian) (German translation: Birkhäuser, 1966)