Archimedean semi-group

A totally ordered semi-group all strictly-positive (strictly-negative) elements of which belong to the same Archimedean class. All naturally ordered Archimedean semi-groups (cf. 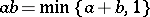Naturally ordered groupoid) are isomorphic to some sub-semi-group of one of the following semi-groups: the additive semi-group of all non-negative real numbers; the semi-group of all real numbers in the interval with the usual order and with the operation ; the semi-group consisting of all real numbers in the interval 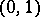and the symbol 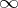with the usual order and with the operations:

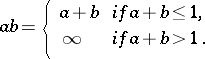The former case occurs if and only if 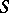is a semi-group with cancellation.

References

[1]	L. Fuchs, "Partially ordered algebraic systems" , Pergamon (1963)

O.A. Ivanova

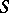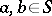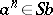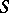A semi-group which satisfies the following condition: For any there exists a natural number such that . If (), the semi-group is called left (right) Archimedean. For commutative semi-groups all these concepts are equivalent. Any commutative semi-group 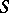is uniquely decomposable into a band of Archimedean semi-groups (and such a decomposition coincides with the finest decomposition of 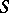into a band of semi-groups). This result may be generalized in a different manner to non-commutative semi-groups [1]. A semi-group 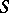with an idempotent is Archimedean (right Archimedean) if and only if it has a kernel 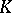and if 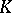contains an idempotent ( 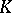is a right group, cf. also Kernel of a semi-group) while the Rees quotient semi-group (cf. Semi-group) is a nil semi-group. Archimedean semi-groups without idempotents are harder to study. A complete description in terms of certain constructions, which is especially clear for semi-groups with a cancellation law [2], [3], was given for the commutative case only.

References

[1]	M.S. Putcha, "Band of 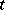-Archimedean semigroups" Semigroup Forum , 6 (1973) pp. 232–239
[2]	A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1–2 , Amer. Math. Soc. (1961–1967)
[3]	T. Tamura, "Construction of trees and commutative Archimedean semigroups" Math. Nachr. , 36 : 5–6 (1968) pp. 255–287