Essential mapping
From Encyclopedia of Mathematics
A continuous mapping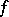 
of a topological space 
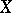into an open simplex 
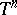such that every continuous mapping 
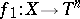that coincides with 
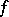at all points of the set 
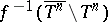is a mapping onto the whole of 
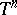. For example, the identity mapping of 
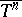onto itself is an essential mapping.
References
| [1] | P.S. Aleksandrov, B.A. Pasynkov, "Introduction to dimension theory" , Moscow (1973) (In Russian) |
Comments
Essential mappings are used to characterize the covering dimension (see Dimension) of normal spaces. A normal space has covering dimension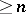 
if and only if it admits an essential mapping onto the 
-dimensional simplex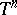 
.
References
| [a1] | R. Engelking, "Dimension theory" , North-Holland & PWN (1978) |
How to Cite This Entry:
Essential mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Essential_mapping&oldid=34342
Essential mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Essential_mapping&oldid=34342
This article was adapted from an original article by M.I. Voitsekhovskii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article