Sperner lemma

If a covering of a closed -dimensional simplex (cf. Simplex (abstract)) consists of closed sets 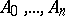that correspond to the vertices of 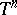in such a way that each face 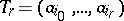of this simplex is covered by the sets corresponding to its vertices, then there exists a point that belongs to all the sets 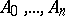. This lemma was established by E. Sperner (see [1]). Sperner's lemma implies that the Lebesgue dimension of the space is . Sperner's lemma can also be used in a proof of Brouwer's fixed-point theorem and Brouwer's theorem on the invariance of domain (cf. Brouwer theorem).

References

[1]	E. Sperner, "Neuer Beweis für die Invarianz der Dimensionszahl und des Gebietes" Abh. Math. Sem. Univ. Hamburg , 6 (1928) pp. 265–272
[2]	P.S. Aleksandrov, B.A. Pasynkov, "Introduction to dimension theory" , Moscow (1973) (In Russian)
[3]	R. Engelking, "General topology" , Heldermann (1989)

Comments

The theorem stated in the main article above is not known as Sperner's lemma in the West, and, in fact, it is not proved in [1].

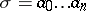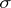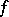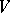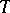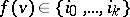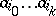Sperner's lemma is the following: Let be an -dimensional simplex, let be a simplicial subdivision of and let be a mapping from (the vertex set of ) to such that whenever belongs to the face of 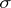. Then the number of -dimensional simplices in 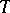on which is surjective is odd.

A mapping like 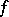above is called a Sperner labelling. Sperner proved this lemma in [1], and obtained as a consequence a somewhat weaker form of the theorem stated in the main article above: his requirement on the sets 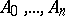was that for every the set 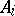should contain the point 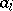and that it should be disjoint from the face of 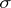opposite to 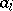. This requirement readily implies the conditions stated in the item.

The theorem stated in the main article above was proved by B. Knaster, K. Kuratowski and S. Mazurkiewicz in [a2], where it was used to prove Brouwer's fixed-point theorem. This form of the theorem is also very useful in infinite-dimensional situations, see [a1], and is sometimes called the KKM-theorem.

Sperner labellings, and Sperner's lemma on subdivisions as given above, have been used to develop effective ways to compute Brouwer fixed points, [a3], and hence such things as equilibria of economics, and, in turn, this development is at the basis of homology methods for the computation of roots of non-linear equations, etc., [a4], [a5].

References

[a1]	J. Dugundji, A. Granas, "Fixed point theory" , PWN (1982)
[a2]	B. Knaster, K. Kuratowski, S. Mazurkiewicz, "Ein Beweis des Fixpunktsatzes für -dimensionale Simplexe" Fund. Math. , 14 (1929) pp. 132–137
[a3]	H.E. Scarf, "The approximation of fixed points of a continuous mapping" SIAM J. Appl. Math. , 15 (1967) pp. 1328–1343
[a4]	E.L. Allgower, K. Georg, "Simplicial and continuation methods for approximations to fixed points and solutions to systems of equations" SIAM Review , 22 (1980) pp. 28–85
[a5]	B.C. Eaves, "A short course in solving equations with PL homotopies" , Proc. SIAM-AMS , 9 , Amer. Math. Soc. & SIAM (1976) pp. 73–143