Kolmogorov integral

A general scheme for constructing an integral, including the Lebesgue–Stieltjes integral, the Burkill integral, the Hellinger integral, etc. Introduced by A.N. Kolmogorov [1]. One considers a directed family of partitions of a space 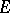of arbitrary nature. A set function 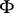(generally many-valued) is defined on the elements of the partition. The sum of the values of this function taken over all elements of the partition gives a many-valued function of the partition. In particular, this sum is a generalization of the Riemann sum 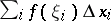where the multi-valuedness is a consequence of the arbitrariness in the choice of the points 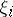on the elements of the partition. The directed limit of the partition functions then defines the Kolmogorov integral 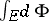. The Kolmogorov integral is considered both for finite and countable partitions. The Kolmogorov integral can be considered for functions with values in a commutative topological group.

References

[1]	A. [A.N. Kolmogorov] Kolmogoroff, "Untersuchungen über den Integralbegriff" Math. Ann. , 103 (1930) pp. 654–696