Principal fundamental solution
From Encyclopedia of Mathematics
A fundamental solution  , defined throughout the space
, defined throughout the space  , of a second-order elliptic equation
, of a second-order elliptic equation
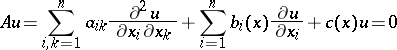 | (*) |
that satisfies the conditions
 |
for certain positive constants  and
and  if
if  .
.
If the coefficients  ,
,  and
and 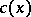 satisfy a Hölder condition on
satisfy a Hölder condition on 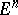 and if the inequality
and if the inequality  is satisfied for some
is satisfied for some  , then a principal fundamental solution exists. If the coefficients of the operator
, then a principal fundamental solution exists. If the coefficients of the operator 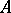 are defined in a certain bounded domain with smooth boundary, then they can be extended to the entire space
are defined in a certain bounded domain with smooth boundary, then they can be extended to the entire space 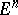 so that a principal fundamental solution will exist for the extended operator.
so that a principal fundamental solution will exist for the extended operator.
References
| [1] | C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian) |
How to Cite This Entry:
Principal fundamental solution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Principal_fundamental_solution&oldid=34113
Principal fundamental solution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Principal_fundamental_solution&oldid=34113
This article was adapted from an original article by Sh.A. Alimov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article