Kronecker-Capelli theorem

compatibility criterion for a system of linear equations

A system of equations

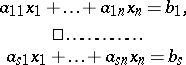is compatible if and only if the rank of the coefficient matrix 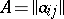is equal to that of the augmented matrix 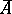obtained from 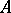by adding the column of free terms .

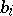Kronecker's version of this theorem is contained in his lectures read at the University of Berlin in 1883–1891 (see [1]). A. Capelli was apparently the first to state the theorem in the above form, using the term "rank of a matrix" (see [2]).

References

[1]	L. Kronecker, "Vorlesungen über die Theorie der Determinanten" , Leipzig (1903)
[2]	A. Capelli, "Sopra la compatibilitá o incompatibilitá di più equazioni di primo grado fra picì incognite" Revista di Matematica , 2 (1892) pp. 54–58
[3]	A.G. Kurosh, "Higher algebra" , MIR (1972) (Translated from Russian)