Harmonics

The simplest periodic functions of the form

These functions are encountered in the study of many oscillatory processes. The number is known as the amplitude, is known as the frequency, is known as the initial phase, and is the oscillation period. The functions are, respectively, the second, third, etc., higher harmonics with respect to the fundamental harmonic. In addition to the harmonics themselves, their sums

(*)

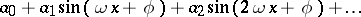are also considered, since a very broad class of functions can be expanded in series of the form (*) in the study of various processes.

Comments

More generally, if is a compact group, is a closed subgroup of and if the regular representation of 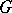on decomposes uniquely into irreducible subrepresentations, then the functions on the homogeneous space belonging to irreducible subspaces of 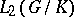are called harmonics, cf. [a1]. For , , 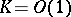one finds the classical harmonics.

References

[a1]	H. Weyl, "Harmonics on homogeneous manifolds" Ann. of Math. , 35 (1934) pp. 486–499
[a2]	E.W. Hobson, "The theory of spherical and ellipsoidal harmonics" , Chelsea, reprint (1955)