Schwarzschild metric

A four-dimensional solution of the vacuum Einstein equations which is static, spherically symmetric and approaches Minkowski space at infinity, found by K. Schwarzschild in 1916.

Comments

With a suitable normalization of the radius function, the conditions above imply the following coordinate representation of the Schwarzschild metric:

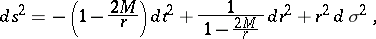where 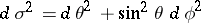is the line element on the standard unit sphere and 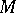is an arbitrary positive constant. This describes a relativistic model of a universe containing a single star only. The source of gravitation in the Schwarzschild universe is the star itself, which does not belong to this space-time.

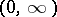The parameter is called the radius function and is assumed to lie in the open interval , with one exception. At the value 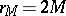, which is called the Schwarzschild radius of the model, the line element given above degenerates. Hence these points have to be removed from the domain where the metric is defined. The part described by 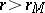is called the exterior Schwarzschild space-time, while for 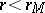one obtains the interior Schwarzschild space-time or the Schwarzschild black hole. Setting the gravitational constant and the velocity of light both equal to , may be identified with the mass of the gravitational source. For example, applying this model to the Sun or the Earth, their Schwarzschild radii are considerably smaller than their geometrical radii.

While for the exterior part the radius function grows in a space-like direction, this is not true for the interior part. There the tangents to the -lines are time-like and the tangents to the 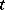-lines are space-like. In this region the terms radius function for and time function for 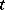are connected with a wrong imagination of these functions. Nevertheless, both solutions satisfy the vacuum Einstein equations. The hypersurface 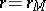separating the interior part from the exterior one only develops from the unified representation of the line element. It cannot be passed by any world line of this model.

Both parts of the Schwarzschild metric can be rediscovered isometrically as open parts of the so-called Kruskal space-time. Here they are separated from each other by a hypersurface 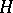, on which the metric remains non-degenerate. This space-time is defined as the warped product 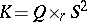, where 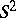carries the standard metric of the unit -sphere and 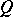is the region in the 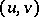-plane defined by 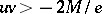, equipped with an indefinite line element 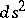as follows:

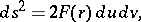where

and where the coordinates and are connected with the Schwarzschild coordinates and 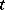as follows:

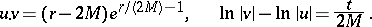In this picture the exterior part is described by the inequalities 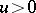and 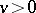, while the interior one (the Schwarzschild black hole) is given by 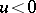and 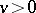. The separating hypersurface 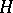is given by 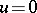, which corresponds to 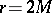and is called the horizon of the black hole.

Any future-directed world line (photon or material particle), starting in the exterior part, has two choices: it remains outside the black hole for all future times or it meets the horizon transversally for some time and then it stays in the black hole for all future times. No future-directed world line can escape from the black hole. Furthermore, the Kruskal extension of the Schwarzschild metric has two additional parts, which can be obtained from the parts described above by a rotation about the angle 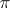in the 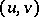-plane. These parts can be considered as a time-reversed Schwarzschild space-time, turning the black hole into a white hole.

The Kruskal space-time is inextendible, i.e. there does not exist any bigger space-time which contains the Kruskal space-time as an open part. But it is not geodesically complete. As well as in the Schwarzschild space-time, there exist causal geodesics for which the natural parameter cannot be extended to all of the real numbers. This implies that there are freely-falling material particles which have bounded proper time only, i.e. they reach the singularity at 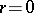after finite proper time.

The Schwarzschild and Kruskal geodesics can be described explicitly using certain functions associated with the corresponding metric. In the time-like (respectively, lightlike) case they represent relativistic orbits of material particles (respectively, photons) in the gravitational field of an isolated star. Considering the shape of these orbits in the case of the solar system, relativistic effects may be observed from the orbits of the inner planets (perihelion advance, light deviation).

A generalization of the Schwarzschild metric is given by the Kerr metric. This is used as a model for rotating black holes. For a detailed discussion of the geometry of these metrics see [a7].

References

[a1]	H. Weyl, "Raum, Zeit, Materie" , Springer (1923)
[a2]	Ya.B. Zel'dovich, I.D. Novikov, "Relativistic astrophysics" , 1. Stars and relativity , Chicago (1971) (Translated from Russian)
[a3]	W. Rindler, "Essential relativity" , Springer (1977) pp. 136–164
[a4]	S. Weinberg, "Gravitation and cosmology" , Wiley (1972) pp. Chapt. 3
[a5]	B. O'Neill, "Semi-Riemannian geometry (with applications to relativity)" , Acad. Press (1983)
[a6]	S.W. Hawking, G.F.R. Ellis, "The large scale structure of space-time" , Cambridge Univ. Press (1973)
[a7]	S. Chandrasekhar, "The mathematical theory of black holes" , Oxford Univ. Press (1983)
[a8]	I.D. Novikov, V.P. Frolov, "Physics of black holes" , Kluwer (1989) (Translated from Russian)