Joachimsthal surface
From Encyclopedia of Mathematics
The surface formed by the orthogonal trajectories of a one-parameter family of spheres with centres on a straight line. If the 
-axis is taken for this straight line, if the 
-coordinates of the centres of the spheres are denoted by 
, and if the radius of the sphere is denoted by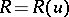 
, then the position vector of the Joachimsthal surface is:
 |
where
 |
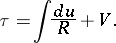One of the families of curvature lines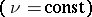 
of the Joachimsthal surface is located in the planes of a pencil. The surface was studied by F. Joachimsthal [1].
References
| [1] | F. Joachimsthal, "Demonstratio theorematum ad superficies curvas spectantium" J. Reine Angew. Math. , 30 (1846) pp. 347–350 |
Comments
References
| [a1] | G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 1 , Gauthier-Villars (1887) pp. 1–18 |
How to Cite This Entry:
Joachimsthal surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Joachimsthal_surface&oldid=33305
Joachimsthal surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Joachimsthal_surface&oldid=33305
This article was adapted from an original article by I.Kh. Sabitov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article