Finitely-presented group

A group on finitely many generators defined by finitely many relations between these generators. Up to an isomorphism, there are countably many such groups. Every set of defining relations between the elements of any finite generating set of a finitely-presented group contains a finite set of defining relations in these generators.

References

[1]	A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian)

Comments

A finitely-presented group is isomorphic to a quotient group 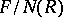, where 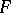is a free group of finite rank and 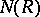is the smallest normal subgroup of 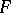containing a given finite subset 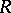of 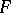(the set of relations).

Some standard references on group presentations are [a1]–[a4].

References

[a1]	H.S.M. Coxeter, W.O.J. Moser, "Generators and relations for discrete groups" , Springer (1984)
[a2]	D.L. Johnson, "Presentations of groups" , Cambridge Univ. Press (1988)
[a3]	R.C. Lyndon, P.E. Schupp, "Combinatorial group theory" , Springer (1977)
[a4]	W. Magnus, A. Karrass, B. Solitar, "Combinatorial group theory: presentations in terms of generators and relations" , Wiley (Interscience) (1966)