Interior differential operator
with respect to a surface 
A differential operator  such that for any function for which it is defined its value at a point
such that for any function for which it is defined its value at a point  can be calculated from only the values of this function on the smooth surface
can be calculated from only the values of this function on the smooth surface 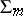 defined in the space
defined in the space  ,
, 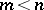 . An interior differential operator can be computed using derivatives in directions
. An interior differential operator can be computed using derivatives in directions 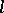 which lie in the tangent space to
which lie in the tangent space to 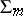 . If one introduces coordinates such that on
. If one introduces coordinates such that on 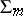 ,
,
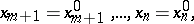 |
then the operator 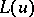 , provided it is interior with respect to
, provided it is interior with respect to 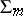 , will not contain, after suitable transformations, derivatives with respect to the variables
, will not contain, after suitable transformations, derivatives with respect to the variables  (the so-called exterior or extrinsic derivatives). For instance, the operator
(the so-called exterior or extrinsic derivatives). For instance, the operator
 |
is an interior differential operator with respect to any smooth surface containing a straight line 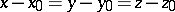 , and with respect to any one of these lines. If the operator
, and with respect to any one of these lines. If the operator 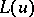 is an interior differential operator with respect to a surface
is an interior differential operator with respect to a surface 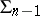 , then
, then 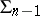 is said to be a characteristic of the differential equation
is said to be a characteristic of the differential equation  .
.
An operator is sometimes called interior with respect to a surface 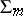 if, at the points of this surface, the leading order of the extrinsic derivatives is lower than the order of the operator.
if, at the points of this surface, the leading order of the extrinsic derivatives is lower than the order of the operator.
Interior differential operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Interior_differential_operator&oldid=33241