Bauer simplex
A non-empty compact convex subset 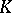 of a locally convex space that is a Choquet simplex and such that the set
of a locally convex space that is a Choquet simplex and such that the set 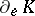 of its extreme points is closed (cf. also Convex hull).
of its extreme points is closed (cf. also Convex hull).
Bauer simplices are also characterized as the compact convex subsets 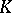 such that every real-valued continuous function on
such that every real-valued continuous function on 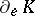 can be extended to a (unique) continuous affine function on
can be extended to a (unique) continuous affine function on  , or, equivalently, for which every point in
, or, equivalently, for which every point in  is in the barycentre of a unique probability measure on
is in the barycentre of a unique probability measure on 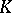 supported by
supported by 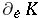 .
.
Such sets have been studied for the first time by H. Bauer [a3]. They were called Bauer simplices in [a1]. See [a1] for their relation with several aspects of convexity theory and potential theory.
More recently (1990s), new connections between them and some general problems in the approximation of continuous functions by positive operators and abstract degenerate elliptic-parabolic problems have been discovered (see, e.g., [a2]).
References
| [a1] | E.M. Alfsen, "Compact convex sets and boundary integrals" , Springer (1971) |
| [a2] | F. Altomare, M. Campiti, "Korovkin type approximation theory and its applications" , W. de Gruyter (1994) |
| [a3] | H. Bauer, "Schilowsche Rand und Dirichletsches Problem" Ann. Inst. Fourier , 11 (1961) pp. 89–136 |
Bauer simplex. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bauer_simplex&oldid=33240