Summation of Fourier series

The construction of averages of Fourier series using summation methods. The best developed theory of the summation of Fourier series is that which uses the trigonometric system. In this case, for functions with Fourier series

the properties of the averages corresponding to the summation method are studied. For example, for the Abel–Poisson summation method, the averages are harmonic functions in the unit disc:

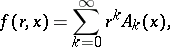while for the summation method of arithmetical averages (cf. Arithmetical averages, summation method of), they are the Fejér sums

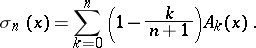Apart from these, the most important in the theory of one-dimensional trigonometric series are the Cesàro summation methods, the Riesz summation method, the Riemann summation method, the Bernstein–Rogosinski summation method, and the de la Vallée-Poussin summation method. Summation methods that are generated by a more-or-less arbitrary sequence of -multipliers

have also been studied.

The summation of Fourier series is used in the following problems.

Representations of functions using Fourier series.

For example, the Abel–Poisson averages , when 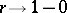, and the Fejér sums , when 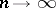, converge to the function 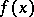at its points of continuity; they converge moreover uniformly if 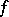is continuous at all points; for every function , these averages converge to the function in the metric of 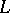and almost certainly. The partial sums of a Fourier series do not possess these properties.

Construction of polynomials with good approximation properties.

The Jackson inequality was established with the help of the summation of Fourier series. In order to solve this problem, as well as using known summation methods, new methods have been proposed, such as the Jackson singular integral and the de la Vallée-Poussin sums (cf. de la Vallée-Poussin sum).

Many properties of functions can be characterized in terms of averages of Fourier series. For example, a function is essentially bounded if and only if there exists a constant such that 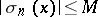for all and .

The summation of Fourier series plays an essential part in the theory of multiple trigonometric series. Thus, instead of spherical partial sums, their Riesz means of sufficiently high order are often used.

Summation of Fourier series is also examined with respect to other orthonormal systems of functions — both concrete systems and classes of systems, for example, orthogonal polynomials, as well as arbitrary orthonormal systems.

References

[1]	N.K. [N.K. Bari] Bary, "A treatise on trigonometric series" , Pergamon (1964) (Translated from Russian)
[2]	A. Zygmund, "Trigonometric series" , 1–2 , Cambridge Univ. Press (1988)
[3]	G.H. Hardy, "Divergent series" , Clarendon Press (1949)
[4]	S. Kaczmarz, H. Steinhaus, "Theorie der Orthogonalreihen" , Chelsea, reprint (1951)
[5]	A.F. Timan, "Theory of approximation of functions of a real variable" , Pergamon (1963) (Translated from Russian)