Graph automorphism
An isomorphic mapping of a graph onto itself (cf. Graph isomorphism). The set of all automorphisms of a given graph forms a group with respect to the operation of composition of automorphisms. The automorphisms of a graph 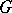 generate a group
generate a group 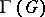 of permutations of vertices, which is called the group (or vertex group) of
of permutations of vertices, which is called the group (or vertex group) of 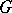 , and a group of edge permutations
, and a group of edge permutations 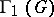 , called the edge group of
, called the edge group of 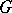 . The edge group and vertex group of a graph
. The edge group and vertex group of a graph 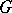 without loops and multiple edges are isomorphic if and only if
without loops and multiple edges are isomorphic if and only if 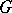 contains not more than one isolated vertex and if none of its connected components is an isolated edge. For each finite group
contains not more than one isolated vertex and if none of its connected components is an isolated edge. For each finite group 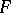 there exists a graph whose automorphism group is isomorphic to
there exists a graph whose automorphism group is isomorphic to 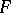 . There also exist permutation groups on a set of
. There also exist permutation groups on a set of  elements which are not the vertex group of any graph with
elements which are not the vertex group of any graph with  vertices. Various types and measures of symmetry of a graph can be related to its automorphisms. A graph with no automorphisms other than the identical one is said to be asymmetric. If
vertices. Various types and measures of symmetry of a graph can be related to its automorphisms. A graph with no automorphisms other than the identical one is said to be asymmetric. If 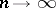 , almost all graphs with
, almost all graphs with  vertices are asymmetric.
vertices are asymmetric.
References
| [1] | F. Harary, "Graph theory" , Addison-Wesley (1969) pp. Chapt. 9 |
Comments
The fact that for a finite group  there is a graph with automorphism group
there is a graph with automorphism group 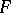 is due to R. Frucht [a3]. A good reference for this and other algebraic aspects of graph theory is [a1]. A related reference is [a2].
is due to R. Frucht [a3]. A good reference for this and other algebraic aspects of graph theory is [a1]. A related reference is [a2].
References
| [a1] | N. Biggs, "Algebraic graph theory" , Cambridge Univ. Press (1974) |
| [a2] | N. Biggs, "Finite groups of automorphisms" , Cambridge Univ. Press (1971) |
| [a3] | R. Frucht, "Herstellung von Graphen mit vorgegebener abstrakter Gruppe" Compos. Math. , 6 (1938) pp. 239–250 |
Graph automorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Graph_automorphism&oldid=33230