Löwner equation

A differential equation of the form

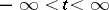where is a real-valued continuous function on the interval . A generalization of the Löwner equation is the Kufarev–Löwner equation:

where 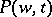, , 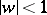, 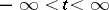is a function measurable in 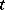for fixed 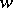and regular in 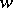, with positive real part, normalized by the condition . The Löwner equation and the Kufarev–Löwner equation, which arise in the theory of univalent functions, are the basis of the variation-parametric method of investigating extremal problems on conformal mapping.

The solution , , 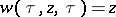of the Kufarev–Löwner equation, regarded as a function of the initial value , for any 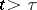maps the disc 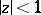conformally onto a one-sheeted simply-connected domain belonging to the disc 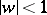. From the formula

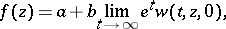by a suitable choice of 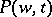in the Kufarev–Löwner equation and complex constants 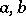one can obtain an arbitrary regular univalent function in the disc . In this way the Löwner equation generates, in particular, the conformal mappings of the disc onto domains obtained from the whole plane by making a slit along some Jordan arc (see [1]–[4]).

The partial differential equation

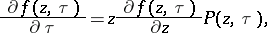which is satisfied by the function

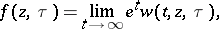is also called the Kufarev–Löwner equation.

The Löwner equation was set up by K. Löwner [1]; the Kufarev–Löwner equation was obtained by P.P. Kufarev (see [5]).

References

[1]	K. Löwner, "Untersuchungen über schlichte konforme Abbildungen des Einheitskreises, I" Math. Ann. , 89 (1923) pp. 103–121
[2]	P.P. Kufarev, "A theorem on solutions of a differential equation" Uchen. Zap. Tomsk. Gos. Univ. , 5 (1947) pp. 20–21 (In Russian)
[3]	C. Pommerenke, "Ueber die Subordination analytischer Funktionen" J. Reine Angew. Math. , 218 (1965) pp. 159–173
[4]	V.Ya. Gutlyanskii, "Parametric representation of univalent functions" Soviet Math. Dokl. , 11 (1970) pp. 1273–1276 Dokl. Akad. Nauk SSSR , 194 : 4 (1970) pp. 750–753
[5]	P.P. Kufarev, "On one-parameter families of analytic functions" Mat. Sb. , 13 (1943) pp. 87–118 (In Russian)
[6]	G.M. Goluzin, "Geometric theory of functions of a complex variable" , Transl. Math. Monogr. , 26 , Amer. Math. Soc. (1969) (Translated from Russian)

Comments

For more information see also Löwner method.