T-ideal

of a free associative algebra

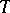A totally invariant ideal, that is, an ideal invariant under all endomorphisms. The set of all polynomial identities of an arbitrary variety of associative algebras over a field (cf. Associative rings and algebras) forms a -ideal in the countably-generated free algebra 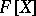, 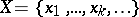. Thus, there exists a one-to-one correspondence between the 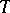-ideals of 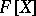and the varieties of associative algebras over 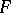. 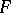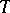If has characteristic , then for every -ideal 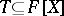there exists a natural number 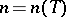such that certain powers of elements of 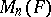are elements of 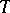, and only they, where 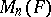is the ideal of identities of the algebra 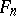of all 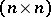-matrices over . 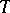In this case a -ideal can also be defined as a (two-sided) ideal that is closed under all differentiations of the free algebra. The quotient algebra 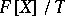is a PI-algebra with 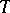as set of polynomial identities. It is called the relatively free algebra (or generic algebra) with 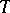-ideal of identities (and is a free algebra in the variety of algebras defined by the identities in 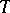). The algebra 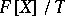has no zero divisors if and only if 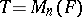for some natural number . 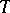Every -ideal 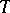of a free associative algebra is primary.

The 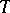-ideals of a free associative algebra on infinitely many generators over a field of characteristic zero form a free semi-group under the operation of multiplication of ideals. In this case a 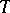-ideal can be defined as an ideal invariant under all automorphisms of the free algebra.

For the question as to whether every -ideal of 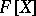is the totally invariant closure of finitely many elements (Specht's problem) see also Variety of rings.

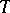-ideals can be defined for non-associative algebras (Lie, alternative and others) by analogy with the associative case.

References

[1]	C. Procesi, "Rings with polynomial identities" , M. Dekker (1973)
[2]	N. Jacobson, "Structure of rings" , Amer. Math. Soc. (1956)
[3]	I.N. Herstein, "Noncommutative rings" , Math. Assoc. Amer. (1968)
[4]	S. Amitsur, "The 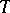-ideals of the free ring" J. London Math. Soc. , 30 (1955) pp. 470–475
[5]	W. Specht, "Gesetze in Ringen I" Math. Z. , 52 (1950) pp. 557–589
[6]	G. Bergman, J. Lewin, "The semigroup of ideals of a fir is (usually) free" J. London Math. Soc. (2) , 11 : 1 (1975) pp. 21–31

Comments

A.R. Kemer has positively solved the Specht problem in the case of characteristic zero (see Variety of rings). He has also introduced the notion of a 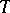-prime ideal, i.e. if 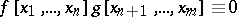(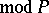) for a 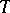-ideal 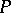, with different variables in 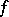and 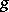, then eithe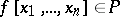r or 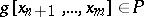. Similarly, for a -nilpotent ideal. He has shown that for every -ideal 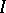there exists a -ideal 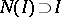such that 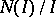is 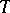-nilpotent and is a finite product of -prime ideals.

References

[a1]	A.R. Kemer, "Solution of the finite basis problem" Soviet Math. Dokl. , 37 (1988) pp. 60–64 Dokl. Akad. Nauk SSSR , 298 (1988) pp. 273–277
[a2]	A.R. Kemer, "Finite basis property of identities of associative algebras" Algebra and Logic , 26 (1987) pp. 362–397 Algebra i Logika , 26 (1987) pp. 597–641
[a3]	E. Formanek, "The polynomial identities and invariants of matrices" , Amer. Math. Soc. (1991)