Optimal quadrature
From Encyclopedia of Mathematics
A quadrature formula giving the best approximation to the integral
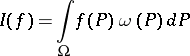 |
for a class  of integrands. If
of integrands. If
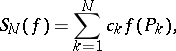 |
then
 |
is called the quadrature error when calculating the integral of a given function, while
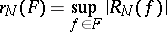 |
is called the quadrature error in the class 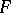 . If a quadrature formula exists such that for the corresponding
. If a quadrature formula exists such that for the corresponding 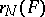 the equality
the equality
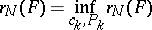 |
holds, then this formula is called the optimal quadrature in this class.
Optimal quadratures have only been found for certain classes of functions which, basically, depend on one variable (see [1]–[3]). Optimal quadratures are also called best quadrature formulas or extremal quadrature formulas.
References
| [1] | S.M. Nikol'skii, "Quadrature formulae" , H.M. Stationary Office , London (1966) (Translated from Russian) |
| [2] | N.S. Bakhvalov, "On optimal convergence estimates for quadrature processes and integration methods of Monte-Carlo type on function classes" , Numerical Methods for Solving Differential and Integral Equations and Quadrature Formulas , Moscow (1964) pp. 5–63 (In Russian) |
| [3] | S.L. Sobolev, "Introduction to the theory of cubature formulas" , Moscow (1974) (In Russian) |
Comments
References
| [a1] | H. Engels, "Numerical quadrature and cubature" , Acad. Press (1980) |
How to Cite This Entry:
Optimal quadrature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Optimal_quadrature&oldid=33175
Optimal quadrature. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Optimal_quadrature&oldid=33175
This article was adapted from an original article by N.S. Bakhvalov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article