Boltzmann equation

An equation in kinetic gas theory, proposed by L. Boltzmann for the determination of the single-particle distribution function of an ideal mono-atomic gas [1]. In dimensionless variables the equation has the form:

(*)

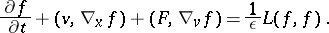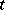Here is the density of the distribution function of the number of particles in the phase space , is the three-dimensional space coordinate, is the velocity, is the time, 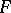is the field strength of the external forces, and is a dimensionless parameter (which is proportional to the ratio between the average distance covered by the particles between successive collisions and the typical scale of the phenomena under consideration). In the simplest case the collision operator has the form:

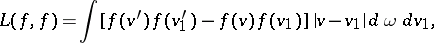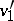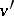where and are the velocities of the molecules before collision, and are the velocities of the molecules after collision, and 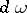is the solid angle element in the direction of the vector .

In deriving the Boltzmann equation it is assumed that the evolution of the function 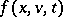is determined by its value at a given moment of time 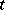and by the pairwise collisions between the gas molecules, and that the time of interaction between two gas molecules during collision is much shorter than the time during which they move independently of each other. From the mathematical point of view the derivation of the Boltzmann equation is based on a certain algorithm which constructs the operator 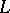in accordance with the well-known laws of motion of two gas molecules which collide with one another.

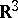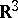In equation (*) the range of variation of the variable is the half-line ; the range of variation of is the entire space ; and the range of variation of is a subspace in ( may coincide with ). In accordance with its physical meaning, the function 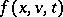should be non-negative and such that

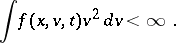The simplest boundary condition on has the form

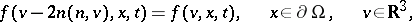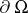where is the normal to . There exist a number of rigorous statements of the Cauchy problem for equation (*).

References

[1]	L. Boltzmann, "Lectures on gas theory" , Univ. California Press , Berkeley (1964)
[2]	N.N. Bogolyubov, "Selected works" , 2 , Kiev (1970) (In Russian)
[3]	S. Chapman, T.G. Cowling, "The mathematical theory of non-uniform gases" , Cambridge Univ. Press (1939)

References

[a1]	C. Cercignani, "Theory and application of the Boltzmann equation" , Scottish Acad. Press (1975)
[a2]	C. Cercignani (ed.) , Kinetic theories and the Boltzmann equation , Springer (1984)