Kirby calculus
Kirby moves
A set of moves between different surgery presentations of a  -manifold.
-manifold.
W.B.R. Lickorish [a3] and A.D. Wallace [a4] showed that any orientable  -manifold may be obtained as the result of surgery on some framed link in the
-manifold may be obtained as the result of surgery on some framed link in the  -sphere.
-sphere.
A framed link is a finite, disjoint collection of smoothly embedded circles, with an integer (framing) assigned to each circle. R. Kirby [a2] described two operations (the calculus) on a framed link and proved that two different framed links,  and
and 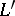 , yield the same
, yield the same  -manifold if and only if one can pass from
-manifold if and only if one can pass from  to
to 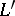 by a sequence of these operations.
by a sequence of these operations.
1) Blow-up: One may add or subtract from 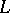 an unknotted circle with framing
an unknotted circle with framing  or
or 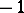 , which is separated from the other circles by an embedded
, which is separated from the other circles by an embedded  -sphere.
-sphere.
2) Handle slide: Given two circles 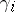 and
and 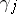 in
in  , one may replace
, one may replace  with
with 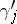 obtained as follows. First, push
obtained as follows. First, push 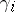 off itself (missing
off itself (missing 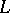 ) using the framing to get
) using the framing to get 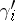 . Then, let
. Then, let 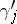 be a band sum of
be a band sum of 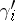 with
with 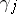 . Framing on
. Framing on 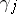 is changed by taking the sum of framings on
is changed by taking the sum of framings on 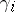 and on
and on 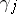 with
with 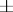 algebraic linking number of
algebraic linking number of 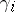 with
with 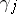 .
.
R.P. Fenn and C.P. Rourke [a1] proved that these operations are equivalent to a 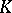 -move, where links
-move, where links 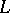 and
and 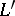 are identical except in a part where an arbitrary number of parallel strands of
are identical except in a part where an arbitrary number of parallel strands of 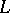 are passing through an unknot
are passing through an unknot  with framing
with framing 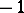 (or
(or 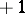 ). In the link
). In the link 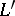 the unknot
the unknot 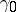 disappears and the parallel strands of
disappears and the parallel strands of  are given one full right-hand (respectively, left-hand) twist.
are given one full right-hand (respectively, left-hand) twist.
References
| [a1] | R.P. Fenn, C.P. Rourke, "On Kirby's calculus of links" Topology , 18 (1979) pp. 1–15 |
| [a2] | R. Kirby, "A calculus for framed links in 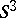 " Invent. Math. , 45 (1978) pp. 35–56 " Invent. Math. , 45 (1978) pp. 35–56 |
| [a3] | W.B.R. Lickorish, "A representation of orientable combinatorial  -manifolds" Ann. Math. , 76 (1962) pp. 531–540 -manifolds" Ann. Math. , 76 (1962) pp. 531–540 |
| [a4] | A.H. Wallace, "Modification and cobounding manifolds" Canad. J. Math. , 12 (1960) pp. 503–528 |
Kirby calculus. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kirby_calculus&oldid=33131