Hammerstein equation

A non-linear integral equation of the type

where and are given functions, while is the unknown function. Named after A. Hammerstein [1], who considered the case where is a symmetric and positive Fredholm kernel, i.e. all its eigen values are positive. If, in addition, the function is continuous and satisfies the condition

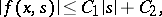where and are positive constants and is smaller than the first eigen value of the kernel 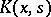, the Hammerstein equation has at least one continuous solution. If, on the other hand, happens to be a non-decreasing function of for any fixed from the interval , Hammerstein's equation cannot have more than one solution. This property holds also if 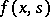satisfies the condition

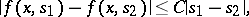where the positive constant is smaller than the first eigen value of the kernel . A solution of the Hammerstein equation may be constructed by the method of successive approximation (cf. Sequential approximation, method of).

References

[1]	A. Hammerstein, "Nichtlineare Integralgleichungen nebst Anwendungen" Acta Math. , 54 (1930) pp. 117–176
[2]	F.G. Tricomi, "Integral equations" , Dover, reprint (1985)
[3]	M.M. Vainberg, "Variational methods for the study of nonlinear operators" , Holden-Day (1964) (Translated from Russian)
[4]	M.A. Krasnosel'skii, "Topological methods in the theory of nonlinear integral equations" , Pergamon (1964) (Translated from Russian)
[5]	N.S. Smirnov, "Introduction to the theory of integral equations" , Moscow-Leningrad (1936) (In Russian)