Instantaneous state

of a homogeneous Markov chain with a countable set of states

A state (say, ) for which the density of the transition probability,

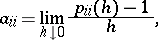where is the probability of transition from 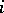to 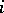in time 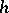, is equal to 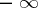. In the opposite case the state 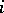is called non-instantaneous, or retarded.

References

[1]	I.I. [I.I. Gikhman] Gihman, A.V. [A.V. Skorokhod] Skorohod, "The theory of stochastic processes" , 2 , Springer (1975) (Translated from Russian)

References

[a1]	D. Williams, "Diffusions, Markov processes, and martingales" , 1 , Wiley (1979)
[a2]	K.L. Chung, "Markov chains with stationary transition probabilities" , Springer (1967)
[a3]	E.B. Dynkin, "Markov processes" , 1 , Springer (1965) (Translated from Russian)
[a4]	D. Freedman, "Brownian motion and diffusion" , Holden-Day (1971)