Steenrod duality

An isomorphism between the 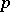-dimensional homology group of a compact subset 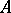of the sphere 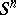and the 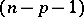-dimensional cohomology group of the complement (the homology and cohomology groups are the reduced ones). The problem was examined by N. Steenrod [1]. When 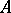is an open or closed subpolyhedron, the same isomorphism is known as Alexander duality, and for any open subset 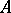as Pontryagin duality. The isomorphism

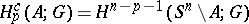also holds for an arbitrary subset 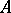(Sitnikov duality); here the 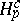are the Steenrod–Sitnikov homology groups with compact supports, and the 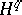are the Aleksandrov–Čech cohomology groups. Alexander–Pontryagin–Steenrod–Sitnikov duality is a simple consequence of Poincaré–Lefschetz duality and of the exact sequence of a pair. It is correct not only for 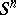, but also for any manifold which is acyclic in dimensions 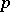and .

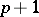References

[1]	N. Steenrod, "Regular cycles on compact metric spaces" Ann. of Math. , 41 (1940) pp. 833–851
[2]	K.A. Sitnikov, "The duality law for non-closed sets" Dokl. Akad. Nauk SSSR , 81 (1951) pp. 359–362 (In Russian)
[3]	E.G. Sklyarenko, "On homology theory associated with the Aleksandrov–Čech cohomology" Russian Math. Surveys , 34 : 6 (1979) pp. 103–137 Uspekhi Mat. Nauk , 34 : 6 (1979) pp. 90–118
[4]	W.S. Massey, "Notes on homology and cohomology theory" , Yale Univ. Press (1964)