Eikonal equation

A partial differential equation of the form

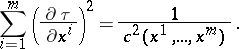Here 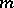is the dimension of the space and is a smooth function bounded away from zero. In applications is the speed of the wave, and the surfaces 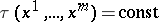are the wave fronts. The rays (see Fermat principle) are the characteristics of the eikonal equation. This equation has a number of generalizations and analogues. In particular, one such generalization is

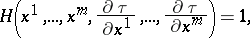where the function 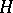is homogeneous of degree 1 with respect to 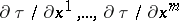and satisfies some additional restrictions. Of considerable interest is the non-stationary analogue

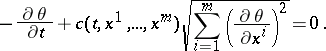This is a special case of the dispersion equations occurring in the theory of wave phenomena, which have the form

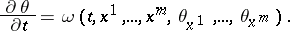Here 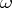is a given function.

The mathematical theory of geometrical optics can be regarded as the theory of the eikonal equation. All forms of the eikonal equation are first-order partial differential equations. The solution of the eikonal equation may have singularities. Their theory is part of that of the singularities of differentiable mappings (see also Hamilton–Jacobi theory; Geometric approximation, and Ray method).

References

[1]	V.M. Babich, V.S. Buldyrev, "Asymptotic methods in problems of diffraction of short waves" , Moscow (1972) (In Russian) (Translation forthcoming: Springer)
[2]	G.B. Whitham, "Linear and nonlinear waves" , Wiley (1974) MR0483954 Zbl 0373.76001

Comments

For a nice account of the theory of geometrical optics see [a3]; geometrical optics and pseudo-differential operator theory are treated in [a2].

References

[a1]	P.R. Garabedian, "Partial differential equations" , Wiley (1964) MR0162045 Zbl 0124.30501
[a2]	M.E. Taylor, "Pseudodifferential operators" , Princeton Univ. Press (1981) MR0618463 Zbl 0453.47026
[a3]	M. Kline, I.W. Kay, "Electromagnetic theory and geometrical optics" , Interscience (1965) MR0180094 Zbl 0123.23602
[a4]	L.B. Felsen, N. Marcuvitz, "Radiation and scattering of waves" , Prentice-Hall (1973) pp. Sect. 1.7 MR0471626