Absolute retract for normal spaces

A topological space such that every mapping of any closed subset of an arbitrary normal space can be extended to the entire space . A direct product of absolute retracts for normal spaces is an absolute retract for normal spaces, as is any retract (cf. Retract of a topological space) of an absolute retract for normal spaces. In particular, the following spaces are absolute retracts for normal spaces: the unit interval ; the -dimensional cube ; and the Hilbert cube . Any two mappings of a binormal space into an absolute retract for normal spaces are homotopic; while a binormal absolute retract for normal spaces is contractible into a point.

Comments

Usually an absolute retract (AR) for normal spaces is defined to be a normal space which is a retract of every normal space in which it is imbedded as a closed subset. A space satisfying the property in the article above is then called an absolute extensor (AE) for normal spaces. One then proves that a space is an (AR) if and only if it is an (AE). Absolute retracts and extensors can be defined for any class of spaces (i.e. not just for normal spaces).

A binormal space is a space 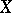for which the product is normal. It can be proved that a space is binormal if and only if is normal and countably paracompact (cf. Paracompactness criteria).

References

[a1]	S.T. Hu, "Theory of retracts" , Wayne State Univ. Press , Detroit (1965)