Antipodes

Diametrically-opposite points on a sphere. Borsuk's antipodal-point theorems apply [1]: 1) For any continuous mapping of the sphere 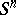into the Euclidean space 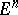there exist antipodes with a common image; 2) Any mapping of the sphere 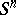into itself in which the images of antipodes are antipodes is an essential mapping.

References

[1]	K. Borsuk, "Drei Sätze über die -dimensionale euklidische Sphäre" Fund. Math. , 20 (1933) pp. 177–190

Comments

The first result mentioned above is known as the Borsuk–Ulam theorem (on antipodes). The following result also goes by the name of Borsuk's antipodal theorem: There is no continuous mapping 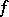of the -ball 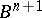into the -sphere 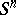such that 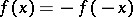, cf. [a1], p. 131.

References

[a1]	V.I. Istrătescu, "Fixed point theory" , Reidel (1981)