Mahler problem
A conjecture in the metric theory of Diophantine approximation (cf. Diophantine approximation, metric theory of) stated by K. Mahler [1]: For almost-all (in the sense of the Lebesgue measure) numbers 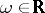 the inequality
the inequality
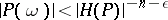 |
has a finite number of solutions in polynomials 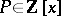 of degree not exceeding
of degree not exceeding  . Here
. Here  ,
,  is a natural number and
is a natural number and 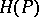 is the maximum modulus of the coefficients of
is the maximum modulus of the coefficients of 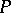 . An equivalent formulation is: For almost-all
. An equivalent formulation is: For almost-all 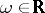 the inequality
the inequality
 |
has a finite number of solutions in integers 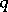 (
(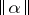 is the distance from
is the distance from  to the nearest integer).
to the nearest integer).
Mahler's problem was solved affirmatively in 1964 by V.G. Sprindzhuk [2]. He also proved similar results for complex and  -adic numbers, and also for power series over finite fields.
-adic numbers, and also for power series over finite fields.
References
| [1] | K. Mahler, "Ueber das Mass der Menge aller 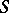 -Zahlen" Math. Ann. , 106 (1932) pp. 131–139 -Zahlen" Math. Ann. , 106 (1932) pp. 131–139 |
| [2] | V.G. Sprindzhuk, "Mahler's problem in metric number theory" , Amer. Math. Soc. (1969) (Translated from Russian) |
Comments
The original paper of Sprindzhuk is [a1].
References
| [a1] | V.G. Sprindzhuk, "A proof of Mahler's conjecture on the measure of the set of  numbers" Izv. Akad. Nauk SSSR Ser. Mat. , 29 (1965) pp. 379–436 numbers" Izv. Akad. Nauk SSSR Ser. Mat. , 29 (1965) pp. 379–436 |
Mahler problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Mahler_problem&oldid=32949