Generalized sequence
net
A mapping of a directed set  into a (topological) space
into a (topological) space 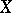 , i.e. a correspondence according to which each
, i.e. a correspondence according to which each 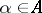 is associated with some
is associated with some 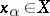 . A generalized sequence
. A generalized sequence  in a topological space
in a topological space  is convergent in
is convergent in  (sometimes one adds: with respect to the directed order
(sometimes one adds: with respect to the directed order  ) to a point
) to a point 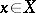 if for every neighbourhood
if for every neighbourhood 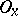 of
of  there exists a
there exists a  such that
such that 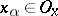 for
for 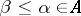 . This is the concept of Moore–Smith convergence [3] (which seems more in conformity with intuitive ideas than convergence based on the concept of a filter). In terms of generalized sequences one can characterize the separation axioms (cf. Separation axiom), various types of compactness properties, as well as various constructions such as compactification, etc.
. This is the concept of Moore–Smith convergence [3] (which seems more in conformity with intuitive ideas than convergence based on the concept of a filter). In terms of generalized sequences one can characterize the separation axioms (cf. Separation axiom), various types of compactness properties, as well as various constructions such as compactification, etc.
Ordinary sequences constitute a special case of generalized sequences, in which 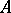 is the set of natural numbers.
is the set of natural numbers.
References
| [1] | J.L. Kelley, "General topology" , v. Nostrand (1955) |
| [2] | M. Reed, B. Simon, "Methods of modern mathematical physics" , 1. Functional analysis , Acad. Press (1972) |
| [3] | E.H. Moore, H.L. Smith, "A general theory of limits" Amer. J. Math. , 44 (1922) pp. 102–121 |
Comments
The phrase "generalized sequence" is hardly ever used in the West; the commonly used terminology being "net" . See also Net (directed set). It should be noted that nets are necessary in the sense that sequences do not always suffice to characterize the various topological properties listed above.
Generalized sequence. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Generalized_sequence&oldid=32935