Dedekind sum
Define 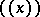 for
for 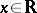 by
by 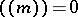 if
if 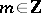 , and
, and 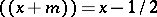 if
if 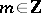 ,
, 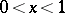 . For integers
. For integers  and
and 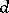 , with
, with  , the Dedekind sum
, the Dedekind sum 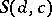 is the rational number defined by
is the rational number defined by
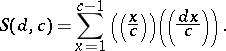 |
R. Dedekind [a1] showed that this quantity occurs in the transformation behaviour of the logarithm of the Dedekind eta-function under substitutions from the modular group. This interpretation leads naturally to the reciprocity relation for Dedekind sums:
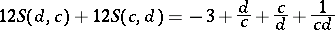 |
if 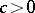 and
and 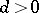 have greatest common divisor
have greatest common divisor 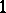 (see also Quadratic reciprocity law). This relation resembles the reciprocity law for power-residue symbols. Several elementary proofs of this relation can be found in [a2]. These proofs exhibit other interpretations of Dedekind sums, related to counting lattice points and Fourier theory (cf. Geometry of numbers; Fourier transform). There are many generalizations, see, e.g., [a3], [a4], [a5], [a6], [a7].
(see also Quadratic reciprocity law). This relation resembles the reciprocity law for power-residue symbols. Several elementary proofs of this relation can be found in [a2]. These proofs exhibit other interpretations of Dedekind sums, related to counting lattice points and Fourier theory (cf. Geometry of numbers; Fourier transform). There are many generalizations, see, e.g., [a3], [a4], [a5], [a6], [a7].
References
| [a1] | R. Dedekind, "Erläuterungen zu den fragmenten XXVIII" H. Weber (ed.) , B. Riemann: Gesammelte mathematische Werke und wissenschaftlicher Nachlass , Dover, reprint (1953) |
| [a2] | H. Rademacher, E. Grosswald, "Dedekind sums" , Math. Assoc. America (1972) |
| [a3] | L.J. Goldstein, "Dedekind sums for a Fuchsian group, I" Nagoya Math. J. , 50 (1973) pp. 21–47 |
| [a4] | L.J. Goldstein, "Dedekind sums for a Fuchsian group, II" Nagoya Math. J. , 53 (1974) pp. 171–187 |
| [a5] | L.J. Goldstein, "Errata for Dedekind sums for a Fuchsian group, I" Nagoya Math. J. , 53 (1974) pp. 235–237 |
| [a6] | U. Dieter, "Cotangent sums, a further generalization of Dedekind sums" J. Number Th. , 18 (1984) pp. 289–305 |
| [a7] | R. Sczech, "Dedekind symbols and power residue symbols" Comp. Math. , 59 (1986) pp. 89–112 |
Dedekind sum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dedekind_sum&oldid=32934