Hurwitz equation

Markoff–Hurwitz equation, Markov–Hurwitz equation

A Diophantine equation (cf. Diophantine equations) of the form

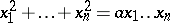(a1)

for fixed , . The case was studied by A.A. Markoff [A.A. Markov] [a1] because of its relation to Diophantine approximations (cf. also Markov spectrum problem). More generally, these equations were studied by A. Hurwitz [a2]. These equations are of interest because the set of integer solutions to (a1) is closed under the action of the group of automorphisms generated by the permutations of the variables , sign changes of pairs of variables, and the mapping

If (a1) has an integer solution and is not the trivial solution , then its -orbit is infinite. Hurwitz showed that if (a1) has a non-trivial integer solution, then 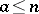; and if , then the full set of integer solutions is the -orbit of 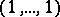together with the trivial solution. N.P. Herzberg [a3] gave an efficient algorithm to find pairs for which the Hurwitz equation has a non-trivial solution. Hurwitz also showed that for any pair there exists a finite set of fundamental solutions such that the orbits are distinct and the set of non-trivial integer solutions is exactly the union of these orbits. A. Baragar [a4] showed that for any there exists a pair such that (a1) has at least fundamental solutions.

D. Zagier [a5] investigated the asymptotic growth for the number of solutions to the Markov equation () below a given bound, and Baragar [a6] investigated the cases .

There are a few variations to the Hurwitz equations which admit a similar group of automorphisms. These include variations studied by L.J. Mordell [a7] and G. Rosenberger [a8]. L. Wang [a9] studied a class of smooth variations.

References

[a1]	A.A. Markoff, "Sur les formes binaires indéfinies" Math. Ann. , 17 (1880) pp. 379–399
[a2]	A. Hurwitz, "Über eine Aufgabe der unbestimmten Analysis" Archiv. Math. Phys. , 3 (1907) pp. 185–196 (Also: Mathematisch Werke, Vol. 2, Chapt. LXX (1933 and 1962), 410–421)
[a3]	N.P. Herzberg, "On a problem of Hurwitz" Pacific J. Math. , 50 (1974) pp. 485–493
[a4]	A. Baragar, "Integral solutions of Markoff–Hurwitz equations" J. Number Th. , 49 : 1 (1994) pp. 27–44
[a5]	D. Zagier, "On the number of Markoff numbers below a given bound" Math. Comp. , 39 (1982) pp. 709–723
[a6]	A. Baragar, "Asymptotic growth of Markoff–Hurwitz numbers" Compositio Math. , 94 (1994) pp. 1–18
[a7]	L.J. Mordell, "On the integer solutions of the equation 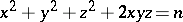" J. London Math. Soc. , 28 (1953) pp. 500–510
[a8]	G. Rosenberger, "Über die Diophantische Gleichung 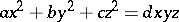" J. Reine Angew. Math. , 305 (1979) pp. 122–125
[a9]	L. Wang, "Rational points and canonical heights on K3-surfaces in 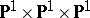" Contemp. Math. , 186 (1995) pp. 273–289