Monodromy operator

A bounded linear operator 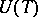which associates to the initial value 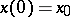the value at time 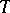of the solution of a differential equation in a Banach space, 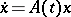, where 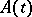is a bounded operator, depending on 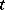, that is continuous and periodic with period : 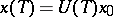. For every solution, 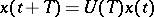. In the finite-dimensional case 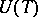corresponds to the monodromy matrix. The position of the spectrum of the monodromy operator influences the existence of periodic solutions of the equation, the behaviour of the solution at infinity, the reducibility of the equation to an equation with constant coefficients, and the presence of exponential dichotomy. 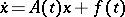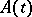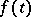The problems of existence and uniqueness of periodic solutions of an inhomogeneous equation with periodic and have also been solved in terms of the spectrum of the monodromy operator.

See also Qualitative theory of differential equations in Banach spaces.

References

[a1]	Yu.L. Daletskii, M.G. Krein, "Stability of solutions of differential equations in Banach space" , Amer. Math. Soc. (1974) (Translated from Russian)